Our "conical fish model" is a mathematical approximation of the shape and volume of a steelhead, which we use to investigate the relationship between the shape of a steelhead and its individual divisor.
|
 |
| Calibrating the Conical Fish Model to Steelhead Shape |
|
|
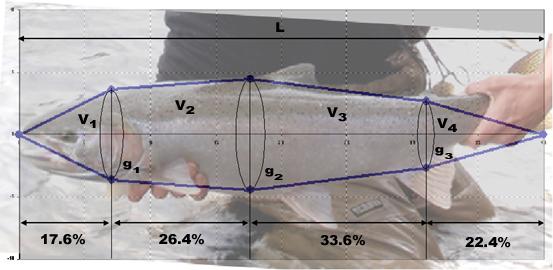
In the diagram above, we roughly approximate the shape of a steelhead by using a cones for the head and tail and two conical mid-sections (technically circular cone frustums). Note that the fractions of length (0.176, etc) are simply rough estimates based on our photographs of several steelhead. (They are not based on in-depth studies.)
To calculate the volume of our "conical fish," we begin by noting that the volume of a cone, V = height * π * radius2 / 3, can be re-written as V = height * girth2 / (12 π), where girth is the circumference of the base of the cone. Applying this substitution to the head-, tail-, and mid-sections yields the following exact equations for the four volumes illustrated.
V 1 = 0.176 L g 12 / (12 π) V 2 = 0.264 L (g 12 + g 22 + g 1 g 2) / (12 π)
V4 = 0.224 L g32 / (12 π) V3 = 0.336 L (g22 + g32 + g2 g3) / (12 π)
|
Summing these volumes yields the following exact formula for the total volume Vc of a conical fish:
|
Vc = V1 + V2 + V3 + V4
Vc = (L / 12 π) (0.440 g12 + 0.600 g22 + 0.560 g32 + 0.264 g1 g2 + 0.336 g2 g3 )
|
Now consider a "base case" conical steelhead: 40 in long (L = 40 in) with girth equal to half its length (g2 = 20 in) and realistically smaller girths at head and tail (g1 = 16.3 in, g3 = 12 in). In this case, the volume Vc of this conical steelhead is 641 in3 (cubic inches) according to the above formula.
If we further assume that our concial steelhead has netural density (neither sinks nor floats), then its weight per unit volume is the same as water: 62.5 lb / ft3. Therefore, according to the following calculations, its weight Wc is 23.2 lb and its individual divisor (divisorc) is 690:
Wc = 641 in3 x (1/123) ft3 / in3 x 62.5 lb / ft3 = 23.2 lb
divisorc = L g22 / Wc = 40 x 202 / 23.2 = 690
|
The fact that divisorc works out to 690 for a "base case" conical steelhead with realistic measurements is nice confirmation that the conical fish model can reasonably represent the weight of actual steelhead (which have an average divisor of 690 according to the data).
Now let's consider a conical steelhead of the same length (L = 40 in), but which is 10% fatter than typical (g2 = 22 rather than 20 in). To realistically represent a stockier fish, let's also increase g1 by 1 inch (g1 = 17.3 rather than 16.3 in) and g2 by about 3/4 in (g2 = 12.73 rather than 12 in). Working through the above calculations yields an individual divisorc of 713 for this stockier fish.
Feel free to program these formulae into a spreadsheet and try as many other sets of realistic numbers as you like. The prediction of the conical fish model is always the same: fat fish (girth g2 greater than half the length) have higher individual divisors than thin fish (girth g2 less than half the length).
Moreover, the above prediction of the conical fish model (i.e. a 10% increase in girth implies a 23 point increase in divisor from 690 to 713) is eerily identical to what we have observed in the data for 115 steelhead.
Based on this correspondence of model results and hard data, we conclude that higher divisors for fat fish and lower divisors for thin ones are not just a theory but are a fact of nature.
|
 |
| Conical Fish Model Predictions |
|
|
|